What is … a Geodesic on a Riemannian Manifold?

This page hosts information on Tobias Pfeiffer & Dror Atariah's talk "What is … a Geodesic on a Riemannian Manifold?" at the "What is …?" seminar. The talk will take place on Friday, January 8, 12:30pm at the BMS Loft in Urania. This talk will help you better understand the BMS Friday talk by Martin Rumpf, which will start at 2pm.

Abstract

What does it mean to "go straight" on a sphere? What is the shortest distance between two points in a space other than the ordinary Euclidean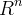 ? These two questions, and many more, are of geometrical nature, and are treated within the framework of differential geometry. The key object that is used is the manifold, which we will define in this talk. We will start from the broadest definition of a topological manifold, and end at the Riemannian one. Then we will give a basic idea and definitions of what a geodesic on a Riemannian manifold is, together with some examples.

Comments

Handout for this talk is available at http://tinyurl.com/whatisgeodesic

-- TobiasPfeiffer - 18 Jan 2010