What is … the direct method in the calculus of variations?

This page hosts information on Beatrice Bugert's talk "What is … the direct method in the calculus of variations?" at the "What is …?" seminar. The talk will take place on Friday, May 27, 12:00pm at the BMS Loft at Urania. This talk will help you better understand the talk by Ulisse Stefanelli, which will start at 2pm.

We will again be ordering delivery pizza. If you would like to order pizza with us, please arrive to the "What is …?" seminar on time.

Abstract

One of the fundamental problems in the calculus of variations consists of finding a function $u$ minimizing the integral functional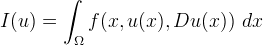

I(u) = \int_\Omega f(x, u(x), Du(x)) \ dx

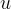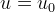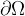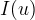over all the functions u satisfying u = u_0 on the boundary \partial \Omega of \Omega, where u_0 is a given function. Euler--often referred to as the founder of the calculus of variations--treated this problem by deducing the so-called Euler-Lagrange equation from the integral functional. He proved that in the case of convex functionals solutions of this equation are already minimizers of I(u). As this method is hard to implement for higher dimensional integrals (i.e., not one dimensional ones), there was a great need to find an alternative method avoiding the Euler-Lagrange equations. It was Riemann who finally succeeded at this task and introduced the so-called direct method in the calculus of variations, which provides the existence of minimizing functions 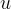u directly from the properties of the functional 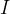I. This talk will give an overview of Riemann's method for convex functionals and show how it has further developed over almost two centuries under the influence of the Italian mathematicians Tonelli and De Giorgi.

Comments